The Truth About “Induced Drag”

Do you really understand what “Induced Drag” is?

For a pilot, the true importance of induced drag is understanding how being on “the back side of the power curve” relates to flight characteristics. Especially in terms of approach to landing.

However, the current way pilots are taught about Induced Drag is flawed, confusing, sometimes incorrect, and often contradictory.

I’ve heard many explanations of Induced drag:

1 – As the angle of attack of a wing increases, the lifting force vector points back more, and this rearward component is Induced Drag
2 – Induced Drag is caused from the Wingtip Vortices creating a greater downwash angle
3 – Induced Drag is caused from the lost energy through Wingtip Vortices
4 – Induced Drag is the drag which increases as a result of lift

So which is it?

The answer is… All of these statements are partially true.

What we have been told to call “Induced Drag” is actually a combination of Pressure Drag, Skin Friction Drag, and “the real Induced Drag”, which all increase with angle of attack.

Let me explain…

PROFILE DRAG

Before considering the forces acting on an entire wing, or airplane, aerodynamicists look at the lift and drag characteristics of a 2-Dimensional (infinitely long) airfoil.

If we sum up the total skin friction and pressure forces acting on the airfoil the results are divided into lift and drag.

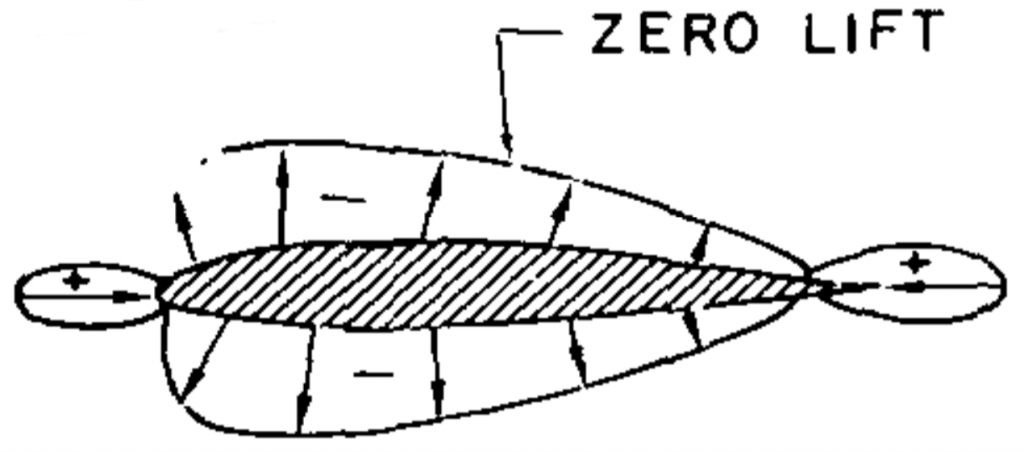
When the airfoil is producing zero lift it is still creating drag.

The drag on just the airfoil is called Profile Drag.

Definition: Profile Drag – “The sum of Skin Friction Drag and Form (or Pressure) Drag. This term is usually used in conjunction with two-dimensional airfoils. It is sometimes also called Section Drag.”

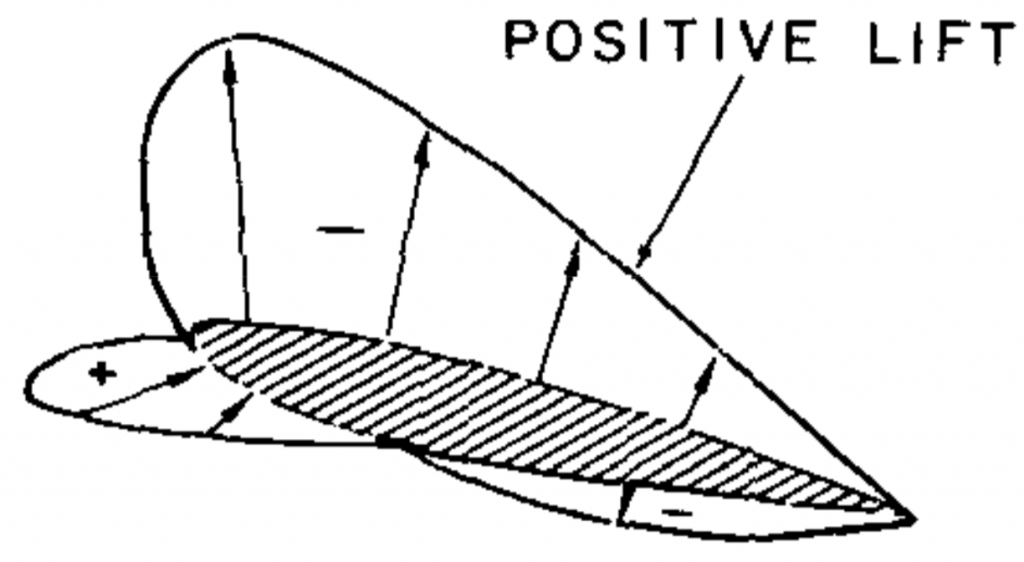
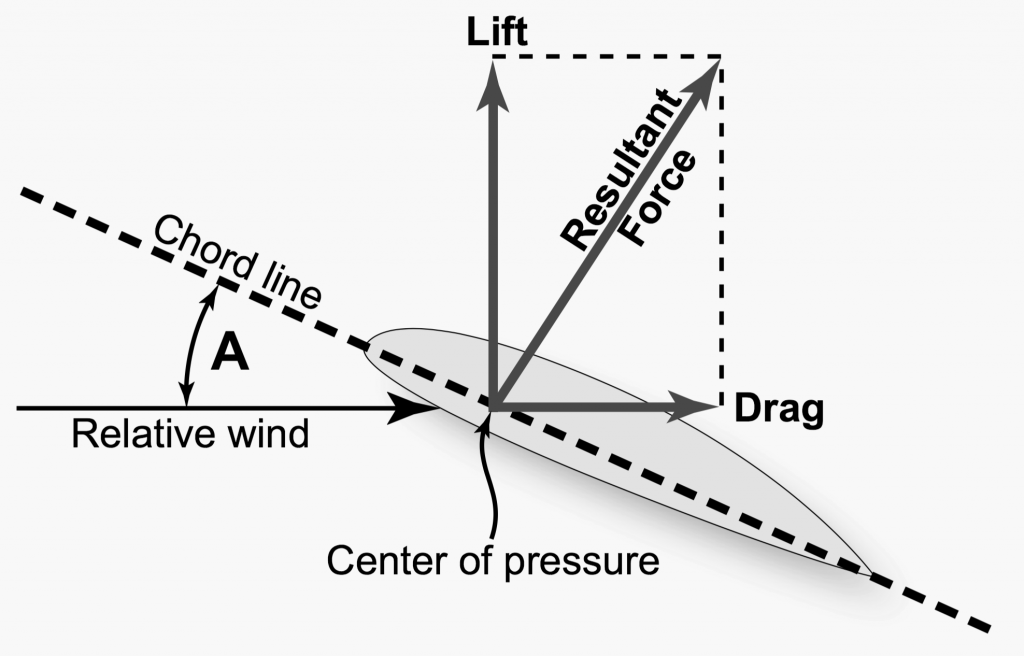
As the angle of attack of the airfoil is increased, the total aerodynamic forces are increased – Shown as “Resultant Force” in the following image.

With greater angle of attack, more lift is produced, and more drag is produced, however this extra drag increase (drag due to lift) would not be called “Induced Drag”, it is simply “Profile Drag”.

In other words the rearward component of the “Resultant Force” on a (2-D) airfoil is called Profile Drag. The equivalent name for this drag on the entire aircraft would be “Parasite Drag”.

Definition: Parasite Drag – “The term used for the profile drag of a complete airplane. It is the portion of the total drag associated with skin friction and pressure drag due to flow separation, integrated over the complete airplane surface. This includes interference drag.”

INDUCED DRAG

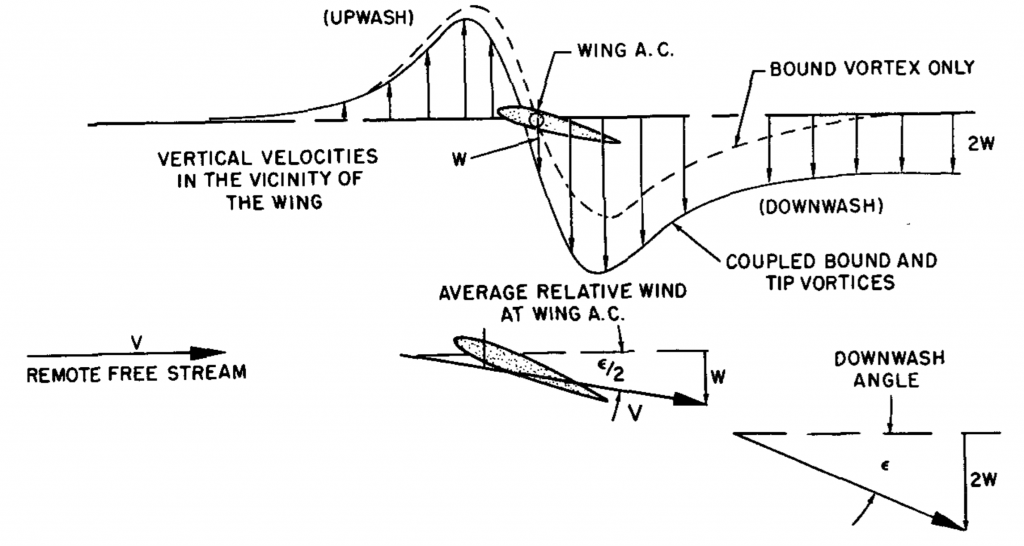
Until this point we’ve only been looking at two dimensional (infinite wingspan) airfoils. When you have a 3-D wing (finite wingspan) you will also have wingtip vortices and “Induced Drag”

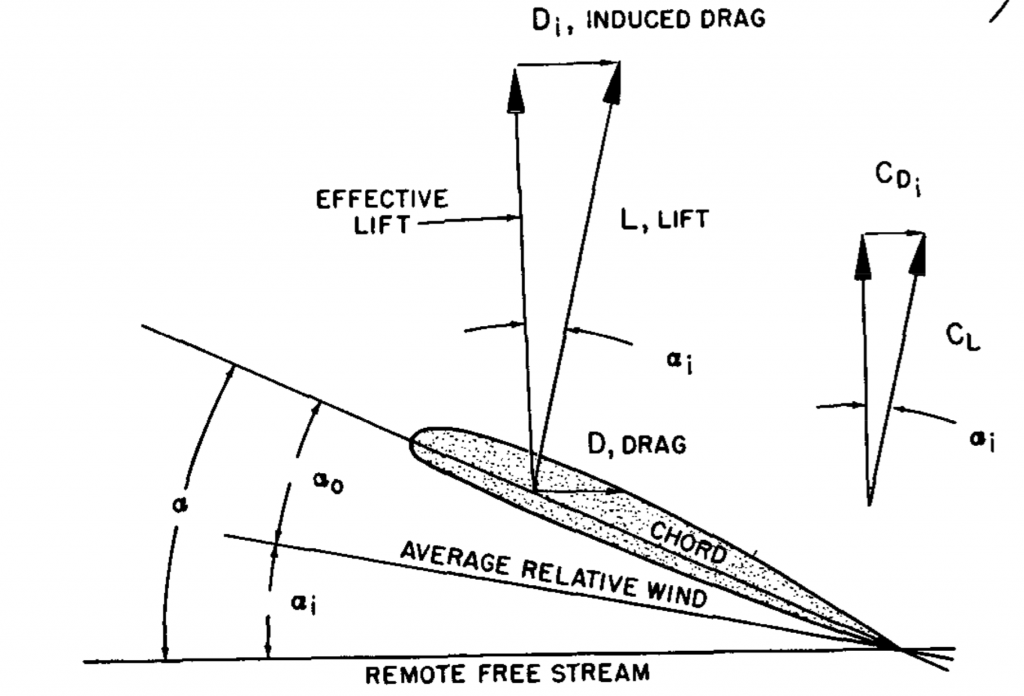
By definition “Induced Drag” is caused from the air spilling over the wingtips causing wingtip vortices, span-wise flow, increased downwash, and inefficiency. It only happens on FINITE wings.

When the air spills over the wings extra drag is formed. This can be modeled mathematically by looking at the local airflow and seeing how the local downwash angle has increased. The vortices shift the local relative airflow in a way which makes the wing “feel” like its at an even higher angle of attack.

This is called the induced angle of attack. This rearward shifting of the resultant force vector (due to wingtip vortices) is Induced Drag.

This is the “real induced drag” as defined by aerodynamicists, and it only happens on 3-D wings because of wingtip vortices.

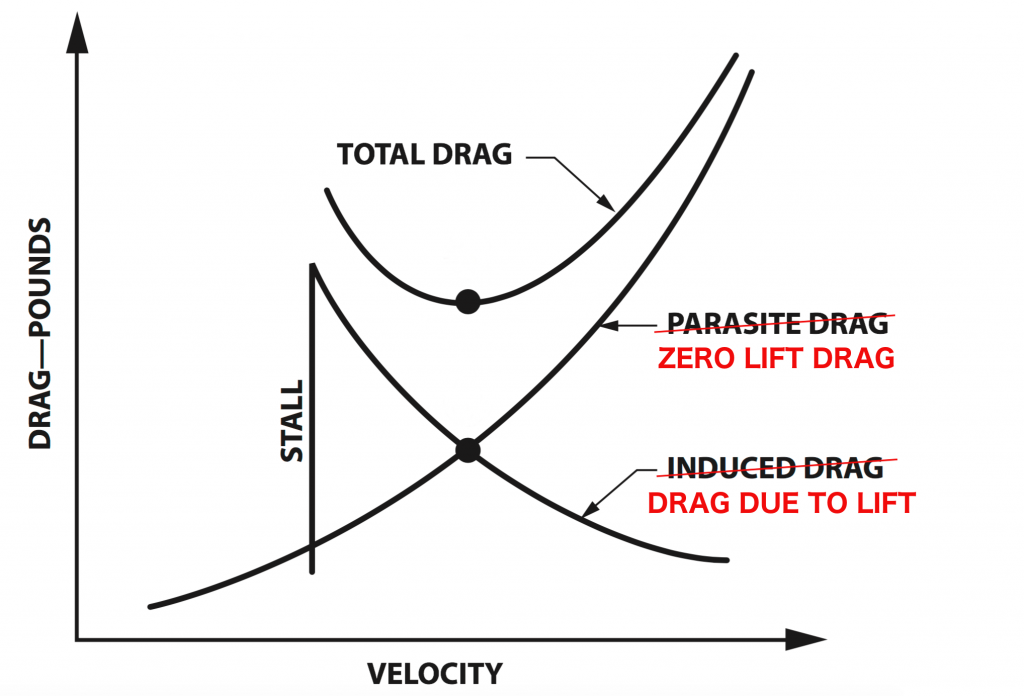
TOTAL DRAG

We can say that total drag is a combination of Parasite Drag, Wave Drag (from approaching the sound barrier) and Induced Drag.

(Total Drag) = (Parasite) + (Wave) + (Induced)

Parasite Drag is the 3-D equivalent of the previously discussed “Profile Drag”

Parasite Drag has a zero-lift component and a component which changes with lift.

Wave Drag also has a zero-lift component and a component changing with lift.

Induced Drag does not have a zero-lift-component and only increases with increased lift.

So:
(Total Drag)

equals

(Parasite Drag from Zero Lift + Parasite Drag Changing With Lift)

plus

(Wave Drag from Zero Lift + Wave Drag Changing With Lift)

plus

(Induced Drag)

Here is where the definition of Induced Drag gets twisted. All of the zero lift drag components can be clumped together as “Zero Lift Drag”.

Also, all of the types of drag which change due to lift can be combined into “Drag Due to Lift”.

Now we can say
(Total Drag) = (Zero Lift Drag) + (Drag Due to Lift)

Which is essentially the famous drag coefficient equation

CD = C_D,0 + KC_L²

Which commonly is describe as (especially in FAA and pilot training literature):

(Total Drag) = (Parasite Drag) + (Induced Drag)

As shown in the total drag diagram:

This image was taken from the FAA Commercial Pilot written test book and is the common convention in teaching pilots. However in proper aerodynamics terms it really makes more sense to re-label the “Total Drag Curve” to the following way.

So in general there are two ways people talk about induced drag.

Pilots usually use the term Induced Drag to describe the total increase in drag as the angle of attack increases. IE – Every drag force which increases with lift.

Engineers tend to use the term Induced Drag to describe purely the drag caused from wingtip vortices.

Sometimes these conventions and definitions get mixed up and people get confused and argue over it. But it really boils down to using whichever definition of “Induced Drag” best fits your needs and audience, however it does help to take a minute and realize the differences between these conventions, and why student pilots might be confused.

NOTE: It’s also been explained to me after writing this post that European pilots and textbooks tend to use to word “Vortex Drag” to avoid the confusion found in the above naming conventions of drag.

The following reiterates what I’ve just said using the Coefficient of Lift and Drag Equations.

$(TotalDrag)=(ParasiteDrag)+(WaveDrag)+(InducedDrag)$